![]() Welcome to my Science blog. These are comments on results that could be (or even are) published.
Welcome to my Science blog. These are comments on results that could be (or even are) published.
Area of a simple irregular polygon in polar coordinates
We discuss here the area of random simple polygons (not self-intersecting or with holes inside). The take-home message is that the area $\mathcal{A}$ of $N$ points defined in the complex plane in polar form as $z_j=re^{i\theta_j}$ with $0\le j\le N-1$ such that $0\le\theta_1\le\cdots\le\theta_N<2\pi$ is: $$\mathcal{A}={1\over2}\operatorname{Im}\sum_{i=0}^{N-1}z_iz_{(i+1)\%N}^*$$ where % means modulo, e.g., $N\%N=0$.
Continuous spectra from planetary nebula: niche history of science
Continuous emission spectra from planetary nebula provide an illustrative case for historians of science of niche discoveries which attracted little attention, despite their possible extremely fundamental nature and needed pursuit.
A mechanism for a perfect single-photon source
The pair correlation function
All radial distribution functions exhibit their first peak at X=1, decreasing monotonically to the first minimum, which is followed by oscillations of diminishing amplitude resembling those of the experimentally determined radial distribution functions of real liquids.—Kirkwood on the pair correlation function, Radial Distribution Functions and the Equation of State of a Fluid Composed of Rigid Spherical Molecules. J. G. Kirkwood, E. K. Maun and B. J. Alder in J. Chem. Phys. 18:1040 (1950).
My last paper[1] (to date) describes a particular shape in the correlation of photons which, we argue, is central to characterizing a perfect single-photon source (see also this twitter thread and this one).
This shape turns out to be, in fact, well-known in condensed matter, physical chemistry and statistical physics. Here you see it from a 1936 paper for mercury (top) and for a macroscopic model of jelly balls suspended in oil (bottom)
Checking your bosonic quantum algebra with Mathematica
I have been working on symbolic computations of bosonic algebra for decades now, see in particular posts on Blog:Notes, especially NormalOrder[] and examples of its use. Here I actualize some observations on the Mathematica piece of code (BosonNormalOrder) which I use to check such results.
Checking your fermionic quantum algebra with Mathematica
Fermionic algebra of the two-level annihilation $\sigma$ and creation $\ud{\sigma}$ operators, e.g., $[\ud{\sigma}\sigma,\ud{\sigma}^\mu\sigma^\nu]=(\mu-\nu)\ud{\sigma}^\mu\sigma^\nu$ as further detailed in our page on this subject and in my Electrodynamic's Wolverhampton Lectures on Physics, are extremely useful to speed up quantum algebra, provided that they are all correct and trustable. This page details the process of their verification.
Our cover of Laser & Photonics Reviews
Our work with Eduardo Zubizarreta Casalengua and Camilo Lòpez Carreño on Conventional and Unconventional Photon Statistics made the cover of Laser & Photonics Reviews (my second cover since PRL's macroscopic condensates). This consecrates Elena and Eduardo's efforts after their key insights made years ago but which we struggled first to write down in a condensed form and then, even more, to publish, which I regard as one confirmation that we understood something very fundamental, very broad, very important and very beautiful. The artwork, which is very Christic, is from Carlos Sánchez Muñoz and represents the basic idea of the paper, which is explained in this blog post, although it is hoped to be self-explanatory for those familiar with the field. It is also simple enough so that there is something for everybody to understand about it.
Overlay of a Mathematica plot with an image
One often wants to compare a printed graph with one's own Mathematical curve. When the curve is fixed, I use Inkscape to do that, with opacity of either one of the images. That's the simplest. If the curve is varying, however, one can use Mathematica's Manipulate to do something similar, on the go. Here's an example
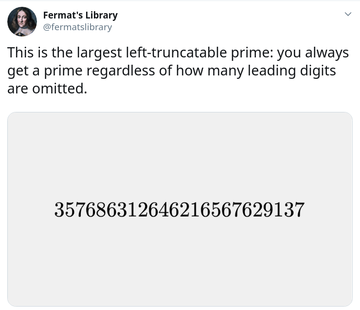
Truncatable primes
@fermatslibrary on twitter posted about an interesting prime:
This is a list of all such primes (there are 4260)
Wading through the Hilbert space
The Hilbert space is a big place. In the words of Douglas Adams (?!):
- Bigger than the biggest thing ever and then some. Much bigger than that in fact, really amazingly immense, a totally stunning size, real 'wow, that's big', time. Infinity is just so big that by comparison, bigness itself looks really titchy. Gigantic multiplied by colossal multiplied by staggeringly huge is the sort of concept we're trying to get across here.
Wavefunction collapse at the Noches de los (muy jovenes) Investigadores (2016)
This year's European Research Night in Madrid (see [1]) was oriented to the very young public (at least this is how the Universidad Autónoma de Madrid understood it [2], and indeed a good fraction of the audience consisted of children).
Our group, that is generously funded by the EU, participated this year again to this event (see here for our last year input), this time with Elena, Carlos & Camilo impersonating herself, Schrödinger & Newton, respectively, to explain the "collapse of the quantum wavefunction" (see what Wikipedia says about that).
Explaining to young people a concept that is still not very well understood by the most experts in the field is a real challenge. This was brilliantly tackled with things that really collapse when probed hard enough: balloons.
Exciting with quantum light
Exciting something with light is such a basic notion, it does not even have a proper subject classification. You would call that "optical excitation", for instance, but it's not something for which you would find a page on Wikipedia. Depending on whom you ask, the closest match could turn out to be something quite different. I would intuitively think of "spectroscopy", which however by far neither fully includes nor is fully included in the original concept.
The o-index
A paper by Dorogovtsev & Mendes in Nature Physics[1] replaces the $h$-index metric (at least $h$ papers cited $h$ times) by the $o$-index, that measures a scientist's impact and productivity through their $\sqrt{hm}$ with $m$ the number of citations to their most quoted paper.
The HOM effect at the Noches de los Investigadores (2015)
Violation of classical inequalities by frequency filtering
Photon correlations are a resource for quantum information processing. If only for the particular case of quantum cryptography: at the single photon level, they can be used for the BB84 protocol. At the two-photon level, they can power the Ekert version[1] that relies on entanglement. It is one requisite if we are to develop a quantum technology to find, engineer and optimize photon correlations.
Emitters of N-photon bundles
Take a light source. Any light source would do, but for convenience, let us consider a laser.
Strong- and weak-coupling: a notion defined at resonance.
The energies of the dressed states of the dissipative Jaynes-Cummings model (refer to this page for notations) read:
