Coherent states may
be of use, so they say,
but they wouldn’t be missed
if they didn’t exist.
Contents |
Quantum States
One popular characterization of quantum states is through Glauber's correlators $g^{(n)}$ (the most famous one being $g^{(2)}$). We provided a nice way to explore the Hilbert space of all quantum states using those as flashlights (see Wading through the Hilbert space).
Fock states
Coherent states
- 🕮Coherent States and Their Applications. J.-P. Antoine and F. Bagarello and J.-P. Gazeau. Springer Proceedings in Physics, 2019. [ISBN: 978-3-319-76731-4] [DOI: 10.1007/978-3-319-76732-1]
Thermal states
Cothermal states
Interpolate between thermal and coherent states.
Negative binomial states
Interpolate between thermal and coherent states.
Glauber-Sudarshan P representation of negative binomial states. K. Matsuo in Phys. Rev. A 41:519 (1990). Template:Fu97a
Binomial states
Interpolate between Fock and coherent states.
Phase states
Number-phase states
Gaussian states
Gaussian states are those which can be created only with displacement operators and squeezing. See Ref. Template:Olivares12a for a tutorial.
Another one-mode definition is:[1]
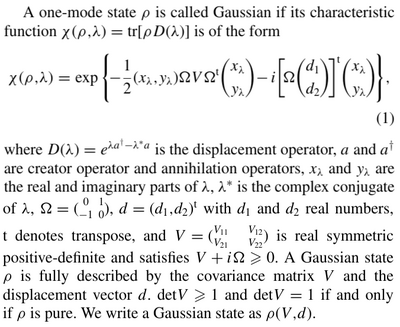
A Gaussian state is pure iff the determinant of the coherence variance matrix = 1.[2][3]