Mathematical Methods II (2013 edition)

This page gathers all the material of the Mathematical Methods II that is specific to the 2013 edition.

Part of the Mandelbrot set.

Computed in class during the "computer session" to answer Problem II of handout 7: the area of the Mandelbrot set in the region $\mathrm{Re}(z)\ge 0\approx2\times 0.2$ (the later figure is computed by pixel counting on the figure here displayed).

This page gathers informations and material related to the course MÉTODOS MATEMÁTICOS II of the Universidad Autónoma de Madrid, 2013, that I taught with Giorgio Cinacchi.

Lectures

• 21.01: Introducing Complex Numbers. (handout 1).
• 22.01: Stereographic projection, Riemann sphere. (lecture by Giorgio Cinacchi)
• 23.01: Complex functions of complex numbers. (handout 2).
• 24.01: Limits and continuity. (handout 3).
• 29.01: Derivatives and analyticity. (handout 4).
• 30.01: Differentiability and Cauchy-Riemann. (handout 5).
• 4.02: Harmonic functions and Laplace equation. (handout 6).
• 5.02: Exponentials, trigonometric functions, hyperbolics and their inverses. (handout 7).
• 11.02: Complex Potentials. (handout 8).
• 12.02: More on conformal mapping. (handout 9).
• 18.02: Integrals in the complex plane. (handout 10).
• 19.02: Line and contour integrals. (handout 11).
• 25.02: The Cauchy-Goursat theorem and its integral forms. (handout 12).
• 26.02: Consequences of the Cauchy theorem. (handout 13).
• 04.03: Advanced topics and further applications.
• 05.03: All questions answered.

• 21.01: Definición. Propiedades algebraicas. Operaciones con números complejos. Representación geométrica de números complejos. Regiones en el plano complejo.
• 22.01: Proyección estereográfica, la esfera de Riemann.
• 23.01: Funciones complejas de variable compleja. Funciones univaluadas y multivaluadas.
• 24.01: Límite de una función. Continuidad.
• 29.01: Derivada de una función. Analiticidad.
• 30.01: Comparación de diferenciabilidad en variable real y variable compleja. Criterios de analiticidad. Condiciones de Cauchy-Riemann.
• 4.02: Funciones armónicas, ecuación de Laplace.
• 5.02: Función exponencial, logaritmo, raíz cuadrada y $n$-ésimas. Funciones trigonométricas, hiperbólicas y sus inversas. Ramas, puntos de ramificación y superficies de Riemann.
• 11.02: Función potencial.
• 12.02: Transformaciones mediante funciones elementales.
• 18.02: Integrales definidas.
• 19.02: Arcos y curvas cerradas. Integrales curvilíneas.
• 25.02: Teorema de Cauchy-Goursat.
• 26.02: Dominios simple y múltiplemente conexos.
• 04.03: Fórmulas integrales de Cauchy
• 05.03: Consecuencias del teorema de Cauchy y teoremas relacionados.

Surveys and results

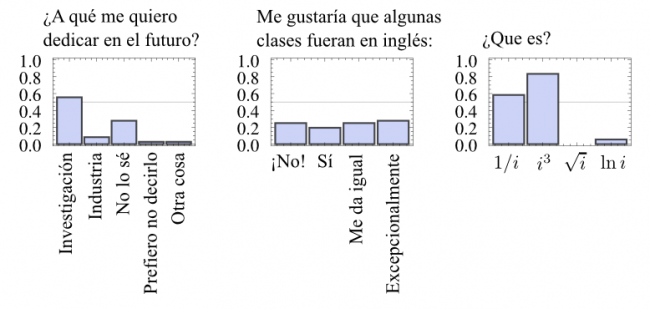
Continuous Evaluation

Exercises distributed in class had to be returned by the following dates:

1. February 4 (verso of handout 4).
2. February 18 (verso of handout 7).
3. March 4 (verso of handout 11).
4. March 2 (this file).
5. April 16 (not yet circulated)
6. April 30 (not yet circulated)

Partial examination

A partial exam has been held on March 12.

• These are the questions.
• These are the answers.

No time constrain (last student left after four hours and 15 minutes). All documents allowed except cross-communication and exiting the room.