Elena & Fabrice's Web
Turning Mathematica lists into hashes
Here is a short hack to turn Mathematica lists into hash tables. Say that you want to apply a function $f$ to the values associated to the following keys:
list1 = {{key1, val1}, {key2, val2}, {key7, val3}}
list2 = {{key1, val4}, {key3, val5}}
list3 = {{key2, val6}}
to have
{{key1, f[val1, val4]}, {key2, f[val2, val6]}, {key3, f[val5]}, {key7, f[val3]}}
The following function does just this:
HashList[op_, lists_] :=
Table[{#[[1, 1]], op @@ #[[2]]}&[
(Transpose[Select[Join @@ lists, #[[1]] == key &]])],
{key, Union[(Join @@ lists)[[All, 1]]]}]
You use it as HashList[f, {list1, list2, list3}]
If you had invoked HashList[Plus, {list1, list2, list3}] you'd get:
{{key1, val1+val4}, {key2, val2+val6}, {key3, val5}, {key7, val3}}
2 January 2025 Depending on what type of function you want to apply, you can op@@ or op@. For instance, to compute error bars on a list such as:
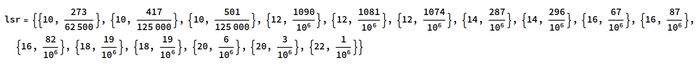
then you could first redefine the function:
HashList[op_, lists_] :=
Table[{#[[1, 1]], op@#[[2]]} &[(Transpose[
Select[Join @@ lists, #[[1]] == key &]])], {key,
Union[(Join @@ lists)[[All, 1]]]}]
Then
lmσ = HashList[Around[Mean[#], StandardDeviation[#]] &, {lsr}]
(note that we use a single list here). This is how it looks (along with a fit in red):
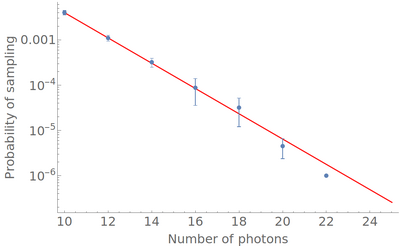
This tells me it'd take about ten million samplings, or about ten days, to get a single 24-photon realization. I could get one 22-photon realization in a day of computation (parallelized over 24 cores). Here it is:
