Disentanglement of Source and Target and the Laser Quantum State. C. Noh and H. J. Carmichael in Phys. Rev. Lett. 100:120405 (2008).  What the paper says!?

Screenshot 20250205 125625.png

On this problem of quantum coherence, Carmichael (and C. Nok) propose that a laser is in the pure coherent state $\ket{\alpha}$.

This is a follow-up of a previous analysis:[1]

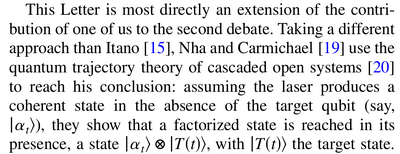
Screenshot 20250205 170054.png

They link their analysis to two broad problems of quantum coherence:

For Molmer, they further link it to J. Javanainen et al.[8]'s prior multiphoton symmetry breaking, which they see as the origin, with Molmer providing «a rather provocative extrapolation» although it seems likely Molmer's approach was independent (and indeed Javanainen's problem is not quite pitch in this way). They also link it to the subsequent problem of continuous variable teleportation.[9][10]

The work is really about the 2nd problem of whether the laser gets entangled to its driving target, and following on Carmichael's previous work,[1] here:

Screenshot 20250205 203121.png

As quoted above, they use the birth-death process of Ref. [11].

They arrive to the interesting conclusion that tracing over some degrees of freedom of the laser, the laser factorizes out from the target it drives and becomes a coherent state. The details of how it comes about deserve further scrutiny. In particular, it is unclear to me whether they have a well-defined phase or if the phase has to be randomized, and thus have no $\alpha$-coherence.[12] Their Eq. (10) doesn't suggest this and they indeed mention «The phase average is the crucial thing». Still, in particular for their discussion of Javanainen & Molmer, it would appear their point is of a pure state, with a definite phase. This would need to be clarified.

They criticize the partition ensemble fallacy (PEF) (first introduced in Ref. [13], see also [14]

fundamental oversight of the quoted ‘‘fallacy’’: the density operator does not provide a complete description of laser light as a quantum field; an infinity of correlation functions do that.

The paper is strangely written. It has several unclear passages, if not outright broken grammar. Already in the abstract, it is unclear what the Authors mean with their «environmental record monitoring laser pump quanta (especially in an abstract! from the text, they mean something like the environment which provides the excitation for the laser, so the active medium which describes the laser). Another confusing sentence is:

We assert that coherent states disentangle the laser source and target not only in their state $\rho(t)$, but as a stochastic process, correlation functions to all orders taken into account.

They apparently mean «correlation function to all orders are [sic] taken into account»

The paper also has a philosophical touch, taking side with particular intepretations of quantum mechanics (here consistent histories):

Screenshot 20250205 182831.png

References

  1. ↑ 1.0 1.1 Decoherence of a two-state atom driven by coherent light. H. Nha and H. J. Carmichael in Phys. Rev. A 71:013805 (2005).
  2. ↑ Optical coherence: A convenient fiction. K. Molmer in Phys. Rev. A 55:3195 (1997).
  3. ↑ Some implications of the quantum nature of laser fields for quantum computations. J. Gea-Banacloche in Phys. Rev. A 65:022308 (2002).
  4. ↑ On the classical character of control fields in quantum information processing. S. v. Enk and H. Kimble in Quantum Inf. Comput. 2:1 (2002).
  5. ↑ Comment on “Some implications of the quantum nature of laser fields for quantum computations”. W. M. Itano in Phys. Rev. A 68:046301 (2003).
  6. ↑ Reply II to “Comment on ‘Some implications of the quantum nature of laser fields for quantum computations’ ”. J. Gea-Banacloche in Phys. Rev. A 68:046303 (2003).
  7. ↑ Reply I to “Comment on ‘Some implications of the quantum nature of laser fields for quantum computations’ ”. S. J. v. Enk and H. J. Kimble in Phys. Rev. A 68:046302 (2003).
  8. ↑ Quantum Phase of a Bose-Einstein Condensate with an Arbitrary Number of Atoms. J. Javanainen and S. M. Yoo in Phys. Rev. Lett. 76:161 (1996).
  9. ↑ Requirement of Optical Coherence for Continuous-Variable Quantum Teleportation. T. Rudolph and B. C. Sanders in Phys. Rev. Lett. 87:077903 (2001).
  10. ↑ Unconditional Quantum Teleportation. A. Furusawa, J. L. Sorensen, S. L. Braunstein, C. A. Fuchs, H. J. Kimble and E. S. Polzik in Science 282:706 (1998).
  11. ↑ Photon statistics of a cavity-QED laser: A comment on the laser-phase-transition analogy. P. R. Rice and H. J. Carmichael in Phys. Rev. A 50:4318 (1994).
  12. ↑ Quantifying the Coherence between Coherent States. K. C. Tan, T. Volkoff, H. Kwon and H. Jeong in Phys. Rev. Lett. 119:190405 (2017).
  13. ↑ Postselected versus nonpostselected quantum teleportation using parametric down-conversion. P. Kok and S. L. Braunstein in Phys. Rev. A 61:042304 (2000).
  14. ↑ https://arxiv.org/abs/quant-ph/0207135
Retrieved from "http://laussy.org/index.php?title=Noh08a&oldid=36136"