Linear model with excitonic interactions
In the previous Section, I have shown how, even for bosonic excitons,
a multiplet structure and some fermionic signatures arise in the
emission spectrum when intra-dot exciton-exciton interactions are
included. In this Section, we will study how these interactions change
the spectral properties of the linear model that we studied in
Chapter 3 in a quantum regime. The results, that we
obtain in the steady state only, are not analytical anymore as in the
previous Section. We describe light-matter interaction in a large QD
including exciton-exciton interactions with the Hamiltonian
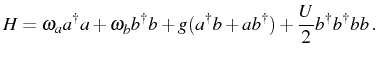 |
(5.10) |
The model couples an HO (photons) with an AO (excitons). In this
Section,
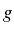
is the unit and
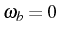
the reference energy. Let us
start by diagonalizing Hamiltonian (
5.10) by
manifolds, in the spirit of Eq. (
2.65). The
structure of eigenenergies at resonance is sketched in
Fig.
5.5 up to a maximum of two
excitations (i.e., up to the second manifold) for three different
cases. First, the bare levels corresponding to noninteracting and
uncoupled (or weakly-coupled) modes (
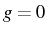
,
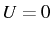
). Second, the
eigenenergies arising from the coupling (
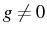
,
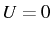
), as we
studied them in Chapter
2 and
3, and
finally, the blueshifted lines that result from including the
interactions (
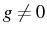
,
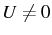
). All the levels but those in the
manifold

, involving only one particle, change with the
interactions because of the excitonic part of their corresponding
eigenvectors. In order to keep track of the excitonic character of
each level, in Fig.
5.6, we plot the
excitonic component of the eigenvectors of manifold
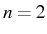
and their
corresponding eigenenergies as a function of the interaction
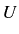
. We
can see that, starting from a situation completely symmetric between
the photonic and excitonic fractions, that the highest level gets more
and more excitonic-like with
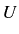
and blueshifts strongly. The other
two energy levels are only slightly affected, as follows from their
more photonic character. This characterization of the levels, which
also depends on the detuning, plays an important role when identifying
the spectral lines, as we show in the following sections.
Figure 5.5:
Energy levels of the eigenstates of the
light-matter coupling Hamiltonian with interactions,
Eq. (5.10) up to the second manifold (two
excitations) at resonance (
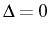 ), left panel for
weak (or no-) coupling (
), left panel for
weak (or no-) coupling (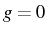 ), central and right
panel in strong-coupling, with right panel also including
interactions
), central and right
panel in strong-coupling, with right panel also including
interactions 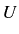 varying on the
varying on the 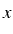 axis. The transitions between
levels account for the spectral features. Red lines correspond to
the vacuum Rabi doublet, that turns into a singlet in WC regime
(transitions in green). Blue lines superimpose to the Rabi doublet
when higher manifolds are probed. Without interactions,
axis. The transitions between
levels account for the spectral features. Red lines correspond to
the vacuum Rabi doublet, that turns into a singlet in WC regime
(transitions in green). Blue lines superimpose to the Rabi doublet
when higher manifolds are probed. Without interactions, 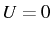 ,
these transitions are not distinguishable in the spectra.
Transitions
,
these transitions are not distinguishable in the spectra.
Transitions
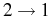 in presence of interactions are plotted
in Fig. 5.7 as a function of the
detuning and
in presence of interactions are plotted
in Fig. 5.7 as a function of the
detuning and 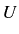 . New qualitative features appear thanks to the
interactions. Black dashed lines are new transitions previously
forbidden, although they remain weak.
. New qualitative features appear thanks to the
interactions. Black dashed lines are new transitions previously
forbidden, although they remain weak.
|
|
Subsections
Elena del Valle
©2009-2010-2011-2012.
![\includegraphics[width=0.6\linewidth]{chap5/brasilia/figure1.ps}](img1373.png)