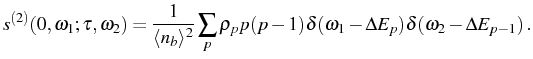Second order correlation function

We already found easily in Sec. 2.5.1 the Hamiltonian spectrum [Eq. (2.89)], consisting of a sum of delta functions at the transition energies $\Delta E_p=\omega_b+U(p-1)$ from each level . In the Hamiltonian dynamics, the mean total population $\ud{b}b$ and the average level populations $\rho_n$ are conserved, and therefore $g^{(2)}(t,t+\tau)$ is independent of time and delay (it stays the same as that of the initial state). The two-frequency/two-time correlator finds also an expression independent of the delay $\tau$ between the two emissions:

$\displaystyle s^{(2)}(0,\omega_1;\tau,\omega_2)=\frac{1}{\langle n_b\rangle ^2}... ..._p \rho_p p(p-1)\delta (\omega_1-\Delta E_p) \delta(\omega_2-\Delta E_{p-1})\,.$

(5.9)

The information in this correlator is clear and expected: if it would be possible to let escape some excitons from such an isolated system, pairs of correlated emissions can only take place, no matter with what delay, when they are consecutive in the manifold structure.

In general, the expressions for the correlators in the purely Hamiltonian dynamics are simple and valid for both positive and negative delays, as the evolution is reversible. It is a subject of future work to study these functions when decay and pump are considered. With only dissipation, analytical formulae probably still exist, but the pump requires again numerical computations.