Covariance of the Schrödinger equation

This page is still largely in progress.

Covariance is a concept that arises with electromagnetism and the special-relativistic understanding that some quantities transform in a peculiar way so as to retain the same equation between different frames of reference. The most famous and popular example is thus Lorentz covariance which is the transformation that rules changes from one inertial frame to the other. The concept can be extended and one can thus speak of, e.g., the covariance of Newton's equation [1]. Lagrange's formulation of mechanics can be seen as a general-covariant formulation (applying to arbitrary changes of variables) [2]. Generally, when referring to an equation, covariance is the property of this equation to retain the same form when changing reference frames, although it involves objects which may change. It is different from invariant which means the equation remains exactly the same.

De Broglie wavelength is not invariant under a Galilean boost but instead transforms accordingly to its interpretation as linear momentum.

Lévy-Leblond has lamented on the lack of textbook description of the problem while others, such as Takagi, say that this is well-known, referring, in to Schiff's quantum mechanics textbook, where the only mention of the fact is made in a problem:

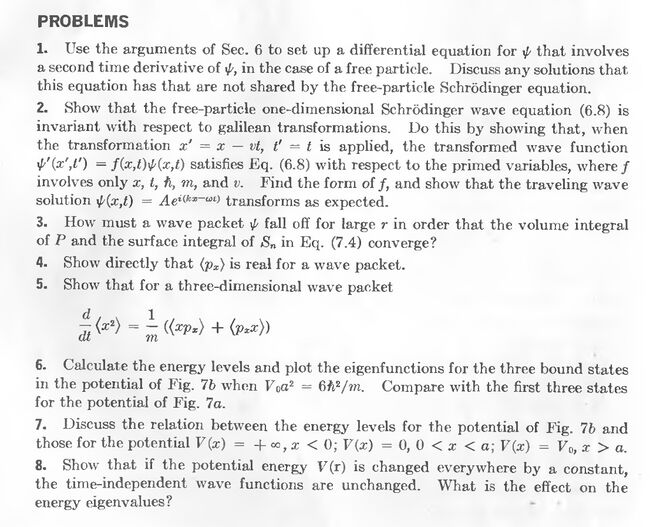
The question of relativistic remnants is an interesting one: this appears in Feynman's proof of Maxwell equations (as reconstructed by Dyson) (Brown says this is spurious).

Template:Brown99a discusses the case of an external field, in particular velocity-dependent ones.

no fully Galilean-covariant theory of a coupled Schrödinger-Maxwell system, where the density and current of the Schrödinger field act as source of the nonrelativistic Maxwell field is possible.

magnetic nonrelativistic limit of Maxwell’s equations studied in 1973 by Le Bellac and Lévy-Leblond.