
The topic itself aims to quantify the usefulness of coherence as a resource. This, thus, makes for "coherence" what was previously done for "entanglement". The Authors themselves pause the question in these terms:
what can be achieved and at what resource cost when the devices that are available to us are essentially classical, that is, they cannot create coherence in a preferred basis.
Several results are indeed almost perfect adaptation from one concept (entanglement) to the other (coherence), e.g., «Just as the maximally entangled state allows for the generation of all quantum operations (Eisert et al., 2000) via LOCC, so does the maximally coherent state allow for the generation of all quantum operations via incoherent operations.»
In this way, various connections are identified between the two. This is clearly a deep and fundamental problem. Many people who studied entanglement in this way are also those who extended this approach to coherence.
The field itself, but more particularly the authoritative texts written on the topic (such as this one), suffer from quite irritating (at least, at first) problems. The main one is that the important concept of "quantum coherence" is not well defined. Quantifying it and relating it to a resource is probably the most practical, clear way to do that. Still, this community managed to make the point particularly confusing by using the terminology of coherence from Glauber's theory of quantum (optical) coherence, to mean basically the opposite, i.e., quantum coherent states for qubits, multi-partite qubits or finite-dimensional systems in general, are superpositions of basis states, i.e., basically, off-diagonal elements in the density matrix. The links is particularly pernicious as the definition is intimately close to that of the quantum-optical coherent state:
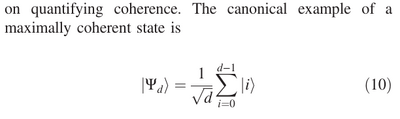
The point is that, here, $d$ is finite. Such states provide universal resources in the sense that applying incoherent operations on them, one can create all other states. The exact opposite appears to be the case with Glauber's coherent states, where those are the Fock states (photons) which are bricks to build everything else. So the quantum-optical coherent states are the resource-less or incoherent ones for the "quantum coherence as a resource". The Authors call that a semantic subtlety:
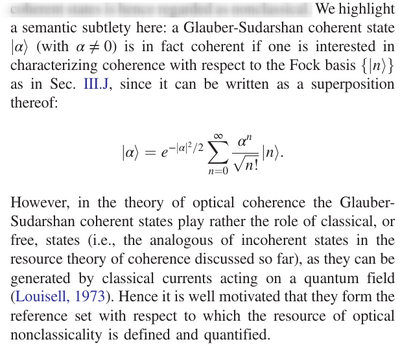
The main discussion from this text for the quantum optician is III. K. 4. Optical coherence and nonclassicality. This is the part which interests me most.
There, the Authors mention that
A general resource theory of nonclassicality, as suggested by Brandão and Plenio (2008), has not been completely formalized yet. In particular, determining the suitable set of free operations for the theory of optical coherence stands as one of the most pressing open questions.
The correspondence in this (quantum-optical, or Glauber) case is not between coherence and entanglement, but between non-classicality and entanglement:
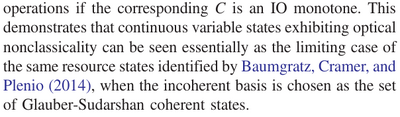
The rest of the work, on non-optical, finite-dimensional Hilbert spaces, which in fact constitutes the bulk and most important part of it, is important but, unfortunately, I find the material annoying in several places for the non-expert, which is a pithy given the importance of the topic.
To start with, the Review of Modern Physics usurped the term "colloquium" (an important and/or large seminar) for some of its articles on trendy or modern topics. The Authors, when lauding their skills in transmitting knowledge, refer to their text as if it was adapted from a previous actual colloquium (what RMP actual invites to do):

I admire their confidence in being accessible yet rigorous, but it is accessible only if you know about Stinespring dilation and Krauss operators. More than a "colloquium", this is a review for people already in the field. For others, the text is painful to read. The text uses the Harvard reference styles, which makes sense for textbooks or encyclopedic works where the focus is on exceptionally important works where each cited piece is cited nominally, because it is in context, e.g., to say that Baumgratz et al. (2014) quantified coherence in a way similar to how Vedral et al. (1997) quantified entanglement. Instead, the work is of a review character, dropping all references at hand on the topic, making a single sentence excruciating to reconstruct from the bomb-cites that clutter it every two words:

Also, given this is a review as opposed to an introduction, the references should be listed by their order of first apparition, not by name, which again makes sense in Harvard encyclopedic style, but not when you list the state-of-the-art. The Authors would say this is the editor's style, and the editor is equally guilty of typesetting such a sore to the eye.
I am also annoyed with their opening statement on what is coherence and its role in Physics:

Electromagnetism appears to belong to today's theories; also, Fresnel's wave optics is not contrasted to ray optics. This contributes to confusion on what they mean by coherence. It carries on like this for a while, e.g., apparently things are often classified as "Quantum Technologies 1.0" (??), and the laser is seen as one-particle coherence!

The laser, as it turns out, is not primarily useful for its "coherence", but for being monochromatic (not what Glauber defines as quantum coherence), intense, and collimated.
In fact, the first thing they should do, is define "coherence", which they don't do!

Not even when they start to explain how to quantify it:

After not defining coherence, the Authors proceed to, at least, define incoherent states. These are states whose density matrix is diagonal, in some agreed-upon basis:

Defining something from its negation is possible, but this still comes too late after many confusing statements on more general definitions of coherence. In their case, coherence is better understood as quantum superpositions of finite number of states (1st-order approximation) which can be exploited as a resource under given sets of operations that do not increase coherence (2nd-order).
After defining incoherent states, they proceed to define "incoherent operations". First, there's no unique definition:

The most important one being:

where $\mathcal{B}(\mathcal{H})$ was defined before Eq. (1) above as «where $\mathcal{B}(\mathcal{H})$ denotes the set of all bounded trace class operators on $\mathcal{H}$.» $\mathcal{H}$ is the Hilbert space, as a "trace class operator", this is a 'linear operator for which a trace may be defined, such that the trace is a finite number independent of the choice of basis used to compute the trace' (according to the Wikipedia), i.e., basically, a matrix with finite trace.
The definition means that incoherent operations cannot generate coherence from incoherent systems, i.e., they cannot create off-diagonal elements from a diagonal density matrix.
This is clearly an important topic and much insights have been provided by the Authors in particular (as researchers), but their exposition of the topic is a bit disappointing.
Quantifying Coherence. T. Baumgratz, M. Cramer and M. Plenio in Phys. Rev. Lett. 113:140401 (2014). Quantifying Entanglement. V. Vedral, M. B. Plenio, M. A. Rippin and P. L. Knight in Phys. Rev. Lett. 78:2275 (1997).