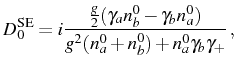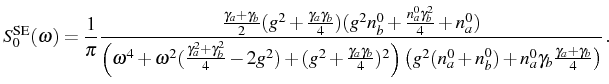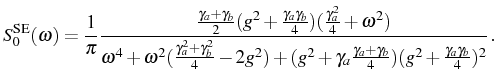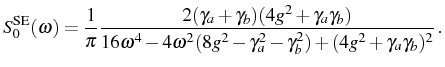Spontaneous Emission
In the most general case of SE, the
![]() coefficient at
resonance,
coefficient at
resonance,
![]() , is a complex number.
If the initial condition further fulfils
, is a complex number.
If the initial condition further fulfils
![]() , it becomes
pure imaginary. Usually [see the work by Carmichael et al. (1989),
Andreani et al. (1999)], the initial states considered are
independent states of photons or excitons (not a quantum
superposition), where indeed
, it becomes
pure imaginary. Usually [see the work by Carmichael et al. (1989),
Andreani et al. (1999)], the initial states considered are
independent states of photons or excitons (not a quantum
superposition), where indeed
![]() . In these cases,
. In these cases,
which yields the following expression for the spectrum:
The SE spectrum of exciton observed in the leaky modes is obtained
from Eq. (3.59) by exchanging the indexes
![]() . We illustrate this with the two particular cases
that follow.
. We illustrate this with the two particular cases
that follow.
The typical detection geometry for the spontaneous emission of an atom
in a cavity consists in having the atom in its excited state as the
initial condition, and observing its direct emission spectrum. In this
case the role of the cavity is merely to affect the dynamics of its
relaxation, that is oscillatory with the light-field in the case of
SC. This case corresponds to ![]() and
and
![]() in
Eq. (3.59) with
in
Eq. (3.59) with
![]() . This gives:
. This gives:
In the semiconductor case, one would typically still have in mind
the excited state of the exciton as the initial condition, but this
time, this is the cavity emission that is probed. The initial
condition is therefore the same as before but without
interchanging ![]() and
and ![]() in Eq. (3.59), which reads in
this case:
in Eq. (3.59), which reads in
this case:
The difference in the lineshape due to the initial quantum state is
seen in Fig. 3.6. The visibility of the line-splitting is
much reduced in the case of an exciton in SC which SE is detected
through the cavity emission, than in the case of a photon, due to a
larger dispersive contribution to the spectrum in the second case. The
reason for such a strong interference term is that the photon is the
most dissipative mode in this example (where
![]() ) and,
therefore, when the system is ``more photonic'' (initiated as a
photon) the overlap between polaritons is more pronounced. With a
polariton as an initial state, only one line is produced.
) and,
therefore, when the system is ``more photonic'' (initiated as a
photon) the overlap between polaritons is more pronounced. With a
polariton as an initial state, only one line is produced.
Again, by symmetry, interchanging
![]() in
Eqs. (3.60) and (3.61), correspond to the SE of
the system prepared as a photon at the initial time and detected in,
respectively, the cavity emission on the one hand
(Eq. (3.60),
in
Eqs. (3.60) and (3.61), correspond to the SE of
the system prepared as a photon at the initial time and detected in,
respectively, the cavity emission on the one hand
(Eq. (3.60),
![]() ), and in the leaky mode
emission on the other hand [Eq. (3.61)]. In the latter case,
the spectrum is invariant under the exchange
), and in the leaky mode
emission on the other hand [Eq. (3.61)]. In the latter case,
the spectrum is invariant under the exchange
![]() .
Fig. 3.6 also hints to the changes brought by the detection
channel (direct emission of the exciton or through the cavity mode).
.
Fig. 3.6 also hints to the changes brought by the detection
channel (direct emission of the exciton or through the cavity mode).
If ![]() or
or ![]() (in which case
(in which case
![]() ), the normalized
spectra do not depend on the nonzero value
), the normalized
spectra do not depend on the nonzero value ![]() or
or ![]() . That
is, one cannot distinguish in the lineshape, the decay of one exciton
from that of two, or more. In the more general case, when
. That
is, one cannot distinguish in the lineshape, the decay of one exciton
from that of two, or more. In the more general case, when
![]() , the peaks can be differently weighted. For instance,
starting with an upper polariton
, the peaks can be differently weighted. For instance,
starting with an upper polariton
![]() (
(
![]() ) gives rise to a dominant
upper-polariton peak (labelled 2 in the above equations, as seen in
the brown dotted line in Fig. 3.6). One can classify the
possible lineshapes obtained for various initial states. For
instance, as we have just mentioned, the normalized spectrum
of
) gives rise to a dominant
upper-polariton peak (labelled 2 in the above equations, as seen in
the brown dotted line in Fig. 3.6). One can classify the
possible lineshapes obtained for various initial states. For
instance, as we have just mentioned, the normalized spectrum
of ![]() as an initial state, is the same whatever the
nonzero
as an initial state, is the same whatever the
nonzero ![]() , which is not unexpected from a linear model. From the
previous statement, the same spectrum is also obtained for a coherent
state or a thermal state of photons, or indeed any quantum state, as
long as the exciton population remains zero. In the same way, the PL
spectrum of the product of coherent states in the photon and exciton
fields,
, which is not unexpected from a linear model. From the
previous statement, the same spectrum is also obtained for a coherent
state or a thermal state of photons, or indeed any quantum state, as
long as the exciton population remains zero. In the same way, the PL
spectrum of the product of coherent states in the photon and exciton
fields,
![]() with
with
![]() , is the same as
that of a polariton state
, is the same as
that of a polariton state
![]() , although both are very
different in character: a classical state on the one hand and a
maximally entangled quantum state on the other.
, although both are very
different in character: a classical state on the one hand and a
maximally entangled quantum state on the other.
Elena del Valle ©2009-2010-2011-2012.